Solutions for Homework #1
- (8 pts. Total)
- 135910
- 11111001112
- 11010101011010112 5463510
- 11058 24516
- (6 pts. Total)
- 100001102 (13410)
- 112 (310)
- 1100001102 (39010)
- (4 pts. Total)
- 1011102
- 1111012
- (10 pts. Total)
- 2310
- -710
- -810
- –910
- 6580910
- (4 pts. Total)
- 0 to 264-1
- –263 to 263-1
- (4 pts. Total)
- 000001102
- 111110112
- (3 pts. Total)
- (1 pt. Total) Prof. Dickey teaches 142 durring 11:30 – 12:30 Mondays
- (1 pt. Total) Mausam’s office hours are 11 – 12 Monday. Nick’s were not announced yet.
- (1 pt. Total) The author of the textbook is a professor at the University of California Berkely
- (1 pt. Total) I hope true!
- (1 pt. Total) The 2nd message was from Alexander Cho.
- (1 pt. Total) The page was: http://www.cs.washington.edu/education/courses/370/98au/admin/Tools/DesignWorks/Tips.html Tip #7 states ‘ "Z" : High Impedance, The probe is not connected to an input signal. So maybe there's a gap in your circuit wiring or the ouput of a module isn't connected to any inputs.’
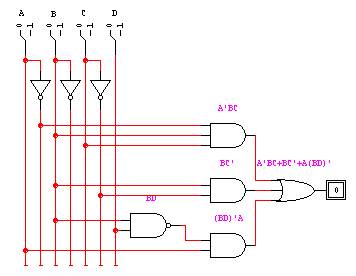
- (5 pts. Total) If we assume that when both switches are off, the light is off, and adopt the encoding 0 = off 1 = on, the following truth table can be derived:
|
A |
B |
Light |
|
0 |
0 |
0 |
|
0 |
1 |
1 |
|
1 |
0 |
1 |
|
1 |
1 |
0 |
This is equivalent to an XOR gate, can can be implemented in gates as
- (6 pts. Total)
- (x+y)*(x+y’) = x+(y * y’) by Distributive Law (8D)
x+(y * y’) = x + 0 by Complementary Law (5D)
x + 0 = x by Identity Law (1)
ð (x+y)*(x+y’) = x
- x*(x+y) = (x+0)*(x+y) by Identity Law (1)
(x+0)*(x+y) = x+(0 * y) by Distributive Law (8D)
x+(0 * y) = x + 0 by Null Law (2D)
x + 0 = x by Identity Law (1)
ð x*(x+y) = x
- (4 pts. Total)
- ID = 0100 0000
So, Size = 1
2n > 1 è n = 1
META-SIZE = 10
I-Tag = 1010 1000 00
Need to add 6 0’s to the end to make an even number of bytes
I-Tag = 1010 1000 0000 0000 è A80016
- ID = 0000 1111 0100 0010 0100 0000
So, Size = 3
2n > 3 è n = 2
META-SIZE = 110
I-Tag = 1101 1000 0111 1010 0001 0010 0000 0
Need to add 3 0’s to the end to make an even number of bytes
I-Tag = 1101 1000 0111 1010 0001 0010 0000 0000 è D87A120016